In nature, we see hyperbolic forms in corals, flatworms, and many other species of reef organisms, such as sponges and kelps. The hyperbolic spaces are also of interest for mathematicians, who are looking to prove the solvability of invariant systems of differential equations in unusual spaces such as these.
Flatworms have a hyperbolic shape.
“Mathematics can be seen as the ‘key’ and milestone to many mysterious problems that are still un-cracked. The theory but also the state-of-art of logical thinking can be used to help other domains of science to progress,” says mathematics researcher Guenda Palmirotta.
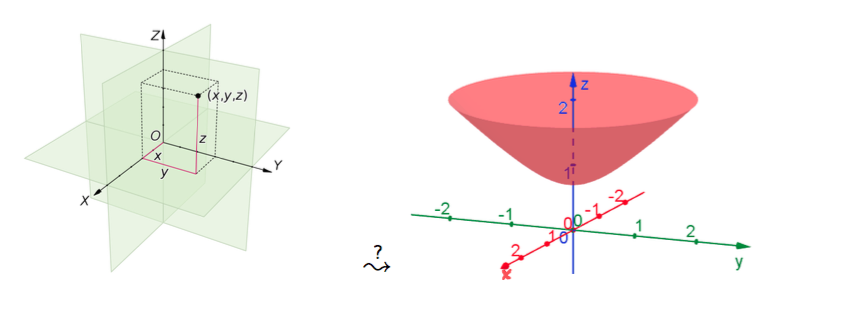
Guenda got the task of proposing a general strategy and prove the solvability of invariant systems of differential equations on some special unusual spaces, such as the hyperbolic plane. It is categorised as a symmetric space and looks like a ‘bowl’ – a shape found abundantly in nature.
This maths problem has been solved for many special cases – but not in general. For example, in the Euclidean situation. Guenda took on this challenge, using elementary approaches in the field, such as functional analysis, complex analysis on several variables and Fourier analysis, in combination with harmonic analysis and representation theory.
An elementary approach to a complex challenge
“By proposing a general strategy, it is possible to prove the problem in every case and not only for special situations as it has been done so far”, Guenda explains.
Guenda & team’s plan was inspired by the Euclidean situation. The idea was to apply the Fourier transform and its properties, rather than exhausting approaches, to present a new method for this challenge.
“We first observed how our colleagues attacked the problem for the Euclidean case. From these observations, we ‘tinker’, in some way, a possible strategy for our non-Euclidean situation.”
However, using this approach, the researchers were confronted to construct solutions of these invariant systems of differential equations satisfying some special and very complicated conditions.
Left the Euclidean situation, right the hyperbolic plane.
Question: Do we have still solvability on symmetric spaces?
RELATED FUNDING
PRIDE
“You need patience, creativity and time”
“Firstly, we simplified these conditions by making them more ‘accessible’. Then, we proved in parallel other important results, which in the end led us to the problem’s solution.
“I compare the situation to a jigsaw puzzle. You need patience, creativity, and time – similar to when working on a research problem. The exception, here, is that we don’t know in advance how many pieces we need to have to complete the puzzle, which is the challenging and exciting part!
“We have found some interesting puzzle pieces: We verified our strategy for specific examples, such as the hyperbolic spaces. Now, the remaining part is to test it on other examples and connect them together to solve the conjecture for the general case and obtain a complete picture.”
Thus, the main challenge remaining is to present a general approach to simplify these difficult special conditions they must meet.
“Depending on the specificity of the situation, in particular for higher dimensions, these conditions could be very difficult to manipulate even after simplification.”
Guenda Palmirotta’s PhD project was part of the FNR PRIDE Doctoral Training Unit (DTU) “Geometric and Stochastic Methods in Mathematics and Applications (GSM)”, coordinated by Gabor Wiese. Guenda defended her PhD on 15 December 2021, from the University of Luxembourg’s Department of Mathematics (supervisor: Prof. Dr. Martin Olbrich). She now works at the Space Weather Office at ESA in Darmstadt, Germany.
Guenda in the control room at ESA.
After completing her PhD, Guenda started work at ESA's Space Weather Office in Germany.
Photos by Guenda Palmirotta
MORE ABOUT GUENDA PALMIROTTA
On her research, peer-to peer
“We need to describe the image of invariant systems of differential equations in symmetric space of non-compact type.
Our strategic approach is to apply the Fourier transform and the Paley-Wiener-Schwartz theorem, to simplify the problem. Following, the idea is to use and adapt several ‘Hörmander-estimation’ results, which are only known in the Euclidean case, for our purposes.
The most difficult part is the verification of the special intertwining conditions coming from the Paley-Wiener-Schwartz theorem.”
On discovering the world of maths
“Since childhood, I was always curious about our universe. After reading many astrophysics books, I understood directly that mathematics plays a major role. It can be seen as the ‘key’ and milestone to many mysterious problems that are still un-cracked. More precisely, the theory but also the state-of-art of logical thinking can be used to help other domains of science to progress. This is one of the many reasons why it intrigued me to study mathematics in the first place.
I chose the department of mathematics at the Université du Luxembourg, because it gave me the possibility to study both points of view, the applied and theoretical aspects. My aim was to deep my theoretical knowledge in harmonic and Fourier analysis theory on symmetric spaces. Spaces, which have a strong connection with our universe.”
On why she loves science
“Science has always been fascinating to me, in particular the universe. It so beautiful but also so mysterious! I am impressed how science provides logic, order, and sense to this territory and even beyond. It allows us to better understand the world around us but also to improve it. Science, especially maths is a tool for life.”
On where she sees herself in 5 years
“I hope that I could use, in a more concrete way, my strong mathematical knowledge to solve astronomy related problems. For the moment, I am looking experience in this field, and I hope to achieve these in the coming years as a research trainee at the European Space Agency (ESA) in the Space Weather Office.”
On mentors with an impact
“Thanks to Prof. Jean-Marc Schlenker, in particular when for suggesting the bachelor topic – he did not only introduce me to the beautiful world of mathematics through programming, but also the research behind it.
I believe that without him, I would probably have ceased after my master studies and would be a high-school math teacher.”
On choosing Luxembourg – home – for research
“When someone asks me why I decided to pursue my studies and research in my home country, I counter reply ‘why not?’.
I did all my studies from Bachelor to Ph.D. at the Université du Luxembourg. I really appreciated the atmosphere and in particular our professors which are very motivated and open to discuss any kind of problems. I was also seduced by the doctoral programme, which proposes young researchers interesting outreach activities and trainings, in order to be prepared for the industrial but also for the academic world in Luxembourg and beyond.”
Guenda, you have participated twice in the Science Festival, which the FNR co-organises with the natur musée. Why was it important for you to participate in this event and what were your experiences?
“It is very important for my colleagues, and me to show that maths is not only a boring class subject of complex formulas, theorems and its proofs, but it is more than that. Actually, maths makes fun, it is everywhere and we can even ‘touch’ it! In other words, with our Science Festival workshops, ‘Unpuzzling maths’ and ‘Jouer, explorer, résoudre’, our aim was to give a completely new image of maths.
“We will never forget how the kids but also adults had an enormous fun playing math games with us, there were so enthusiast and focused on their game that it was hard for them to move to the next stand!”
Guenda Palmirotta at her PhD defense.
"The important day finally came, December 15 2021, my PhD defense day. Attending with excitement my jury and the start of my defense."
Guenda's PhD jury committee, from left to right: Prof. Schlenker (president of the jury), Prof. Pedon (Uni Reims), Guenda, Prof. Mehdi (Uni Lorraine), Prof. Olbrich (supervisor) and Prof. van den Ban (Uni Utrecht).
"This is a screenshot of my defense presentation. In this slide, I explained the strategy how I attacked the problem. It summed up perfectly all steps and important theorems in a sketch."
About Spotlight on Young Researchers
Spotlight on Young Researchers is an annual FNR campaign where we shine a Spotlight on early-career researchers across the world with a connection to Luxembourg. Nearly 100 features have been published since the first edition in 2016.
